Man-made composite materials have generated a wealth of studies in the community of wave physics over the past 20 years, as they can have properties that cannot be found in natural materials. It is now well accepted that the properties of these propagation media stem from two distinct origins : the ordered or disordered spatial distributions of their constituents, and the resonant or non-resonant nature of their unit cells. When waves propagate in a composite media that has a structural order, they can undergo multiple scattering, which leads to frequency bands of permitted and prohibited propagation. These prohibited propagation bands (defined as band gaps), are analogous to electronic band gaps in natural crystals (Kittel, 1996). These materials have a typical spatial scale comparable to the wavelength of the frequencies of interest. They are termed photonic crystals in electromagnetics and phononic crystals in acoustics, and they constitute powerful tools to shape the flow of waves in various ways (Yablonovitch, 1987 ; Joannopoulos et al, 2008 ; Deymier, 2013).

On the other hand, composite materials made out of resonant elements (also known as locally resonant media) owe their macroscopic properties to the dispersive nature of their unit cells (Liu et al, 2000 ; Pendry et al, 1999, 2000 ; Engheta and Ziolkowski, 2006). They can be organized at a scale smaller than the wavelength, such that they belong to the family of metamaterials. In locally resonant metamaterials, the resonators are typically sub-wavelength, i.e., of a size smaller than the propagated wavelength (Liu et al, 2000 ; Lemoult et al, 2011a), and they are arranged over a distance that allows coupling between them. In general, the metamaterial properties can be interpreted through interference between the propagating and scattered waves. This interference leads to hybridization between the local resonance and the incident waves that propagate in the host medium (Lemoult et al, 2013). The spatial organization of the material is no longer relevant, and band gaps can be observed whether it is ordered or disordered (Kaina et al, 2013).

The width and efficiency of band gaps in locally resonant metamaterials depend upon both the spatial density of the resonators and the quality factor of the resonance. Indeed, in the limit of small resonators compared to the wavelength, the smaller the resonator, the higher its quality factor. This justifies why locally resonant metamaterials classically support band gaps limited to narrow bandwidths. To overcome this fundamental limitation, a dimension of the physical space can be sacrificed with the creation of a uni-axial metamaterial, which consists of resonators that are small compared to the wavelength in two dimensions, while this restriction is relaxed for the third dimension. One example is the so-called wire medium in electromagnetism, which has been used to control and/or focus waves below the diffraction limit (Belov et al, 2006 ; Lemoult et al, 2011b). In acoustics, a collection of narrow, but long, pipes was recently used to form a super lens (Zhu et al, 2010). Although limited so far to three-dimensional (3D) metamaterials, this approach is perfectly suited to seismic surface waves that interact with a collection of vertical trees, as will be found in any forest at the geophysics scale.

One of the applications of metamaterials is the possibility to control and manipulate waves at different wavelength scales. In practice, the quest for an invisibility cloak that can be used to conceal an object from incident wavefields started a few years ago in the field of optics (Pendry et al, 2006 ; Leonhardt, 2006). It did not take too long for the concept to cross over into the realm of solid mechanical waves, such as fully coupled shear and longitudinal waves that require either elastic media described within the framework of modified Willis equations (Milton et al., 2006) or Cosserat media described by transformed Navier equations displaying an asymmetric elasticity tensor (Brun et al., 2009).

It was theoretically shown by Farhat et al. (2009), and with subsequent experimental demonstration by Stenger et al. (2012), that a geometric transform in the Kirchhoff-Love plate equation leads to anisotropic Young’s modulus and isotropic density that both vary spatially. Such metamaterial parameters make possible an invisibility cloak for flexural waves (in a plate). One constraint is that strong anisotropy of the Young’s modulus is required on the inner circular boundary of the elastic cloak, as well as a vanishing density, to force the propagation of flexural waves around a cloaked region (i.e., a disk surrounded by a metamaterial ring ; see section WP1.1 Fig. 2). However, the cloak parameters in Farhat et al. (2009) can also be interpreted in terms of an anisotropic effective index that has a decreasing entry toward the center of the cloak, and a large entry along the direction of its circular boundary.

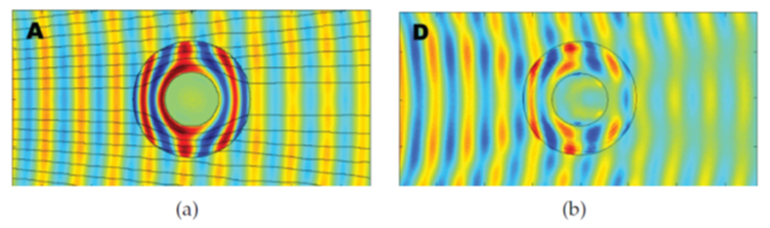
When considering surface waves in plates, it was shown that the Kirchhoff-Love equation that governs their propagation dramatically simplifies the design of a mechanical cloak for these waves, as it retains its form under coordinate changes unlike for the Navier (Farhat et al., 2009). Some experiments have indeed demonstrated cloaking over a broad range of frequencies for Lamb waves in a microstructured thin plate (Stenger et al., 2012).

Finally, another application of metamaterials is the possibility to control and manipulate waves at different wavelength scales, and for example to create a superlens, and/or generate images with super-resolution. The concept of a superlens (Pendry, 2000 ; Fang et al, 2005 ; Taubner et al, 2006 ; Smolyaninov et al, 2007) recently revealed the possibility of obtaining an image of a source without being limited by the diffraction limit. Indeed, such a superlens has the remarkable capacity to amplify the evanescent near-field wavefield contribution, which is of great interest for near-field microscopy, for example. The superlens exploits the negative refraction property of a Veselago lens (Veselago, 1968), which can be found in natural materials for particular frequency bands (Bramhavar et al, 2011) or can be tuned using a metamaterial (Smith et al, 2004 ; Soukoulis et al, 2006). This tuning allows modification of the effective properties of a medium using sub-wavelength resonators. For example, split-ring resonators (Pendry et al, 1999) are used for electromagnetic waves, which provides the opportunity to obtain an effective medium that has both negative permeability and permittivity. Based on the idea of a locally resonant medium, the possibility to break the diffraction limit from the far-field was first reported with electromagnetic waves using uni-axial resonators (Lerosey et al, 2007, Lemoult et al, 2010). In such a configuration, the sub-wavelength nature of the metamaterial is due to the number of resonators within one wavelength in a 2D plane perpendicular to the resonator axis. When transposed to elastic waves, uni-axial sub-wavelength resonators were shown to provide great complexity in terms of the dispersion of Lamb waves propagating in a thin plate (Khelif et al, 2010 ; Oudich et al, 2011 ; Xiao et al, 2012). In particular, Rupin et al. (2014) experimentally demonstrated the presence of sub-wavelength and supra-wavelength modes.

Updated on 5 octobre 2016